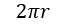The Electric Flux:
Electric Flux Law: (considering that the electric field is regular)
The area vector:
The area vector has a magnitude and a direction that is always perpendicular to the area towards the outside.
The Cases of the angle:
|
|
|
|
If the electric field is perpendicular to the area vector |
|
|
If the electric field is parallel to the area vector (Same direction) |
 |
|
If the electric field is parallel to the area vector (opposite direction) |
|
|
If the electric field is no perpendicular nor parallel to the area vector |
 |
Electric Flux Law: (considering that the electric field is irregular)
Gauss's law:
It describes the relationship of electric flux (through a closed surface) to the positive charge (within that closed surface).
Notes:-
1- The previous law means that the electric flux through any closed surface around a positive charge is not affected by the shape of that surface.
2- The direction of the electric field is perpendicular to the area, and parallel to the area vector (in the same direction).
Conditions for applying Gauss's law:-
1- The charge is evenly distributed
2- The surface is closed (ball, cylinder ...)
Important laws
- The circumference of a circle:
- The area of a sphere:
- The volume of a sphere:
Calculating the Electric Field through a Gaussian surface:-
Case 1: if the Gaussian surface is a ball: - The electric field at any point is
Case 2: if the Gaussian surface is a cylinder: - The electric field at any point is
